How to Draw a Line of Best Fit in Maths
Physics Practical Skills Part 4: Drawing graphs and lines of best fit
In part 4 of the Physics Skills Guide, we explain how to draw a line of best fit correctly in Physics Practicals. Read the post to learn about dos and don'ts of drawing a line of best fit.
Introduction to Drawing Graphs and Lines of Best Fit
In this Guide, we explain the importance of scientific graphs in Physics and how to draw scientific graphs correctly including lines of best fit. This is a really important Physics skill and you'll need to master it if you want to ace your next Physics Practical exam.
In this article we discuss:
- Scientific graphs
- Drawing the line of best fit
- Common mistakes with drawing lines of best fit
Scientific Graphs
What are scientific graphs?
A graph is a visual representation of a relationship between two variables, x (the independent variable) and y (the dependent variable).
Graphs make it easy to identify trends in data that you have collected and can be analysed in order to perform a calculation or a measurement, related to addressing the aim of an experiment.
How to draw a scientific graph
| Step | Action | Detail |
| 1 | Identify the variables |
|
| 2 | Determine the variable range |
|
| 3 | Determine the scale of the graph |
|
| 4 | Number and label each axis |
|
| 5 | Plot the data points |
|
| 6 | Draw the graph |
|
| 7 | Provide a descriptive title |
|
In Year 11 and 12 Physics, the trends that you will investigate are mostly linear, or can be converted into linear graphs, and, hence, you'll be required to draw a line of best fit.
Drawing the line of best fit
An example of a correctly drawn line of best fit is shown below, along with an incorrect one:
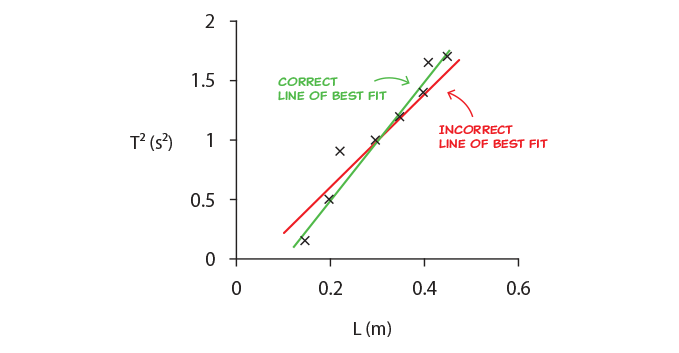
How to draw a line of best fit
To draw the line of best fit, consider the following:
- Outliers must be ignored.
- The line must reflect the trend in the data, i.e. it must line up best with the majority of the data, and less with data points that differ from the majority.
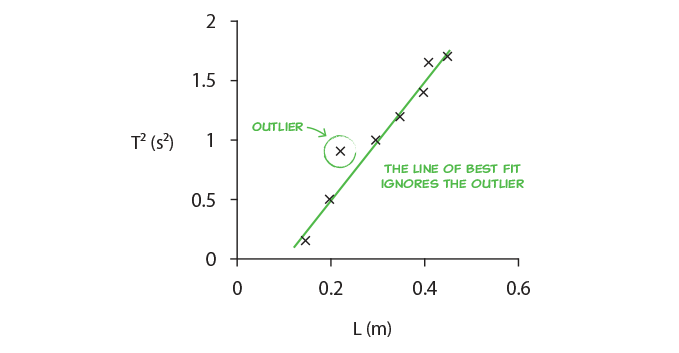
- The line must be balanced, i.e. it should have points above and below the line at both ends of the line.

- DO NOT force the line to pass through any specific data points, or to pass through zero. The purpose of the line of best fit is to reveal the trend of all the data.
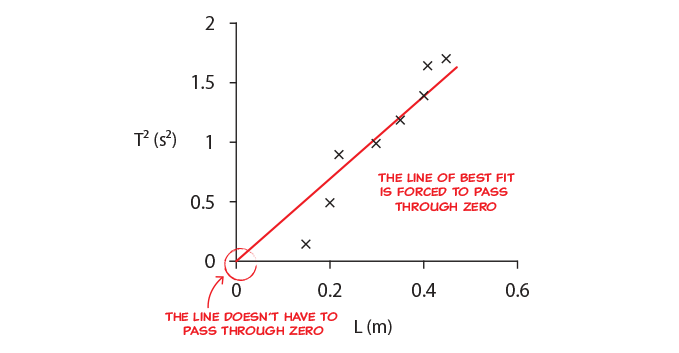
Why do we need to draw a line of best fit?
The main reasons for drawing a line of best fit are to:
- Reduce the effect of both systematic and random error and thus make the experiment more accurate and more reliable.
- Find and use the gradient of the line of best fit to determine an unknown in the experiment. Usually determining this unknown addresses the aim in some way
How does the line of best fit reduce experimental errors?
By drawing the line of best fit we are looking for the strongest trend in the data, and in this way reduce the effect of random errors. If we then use the gradient of the line of best fit we look at differences in values rather than absolute values, which reduces or eliminates the effect of systematic errors.
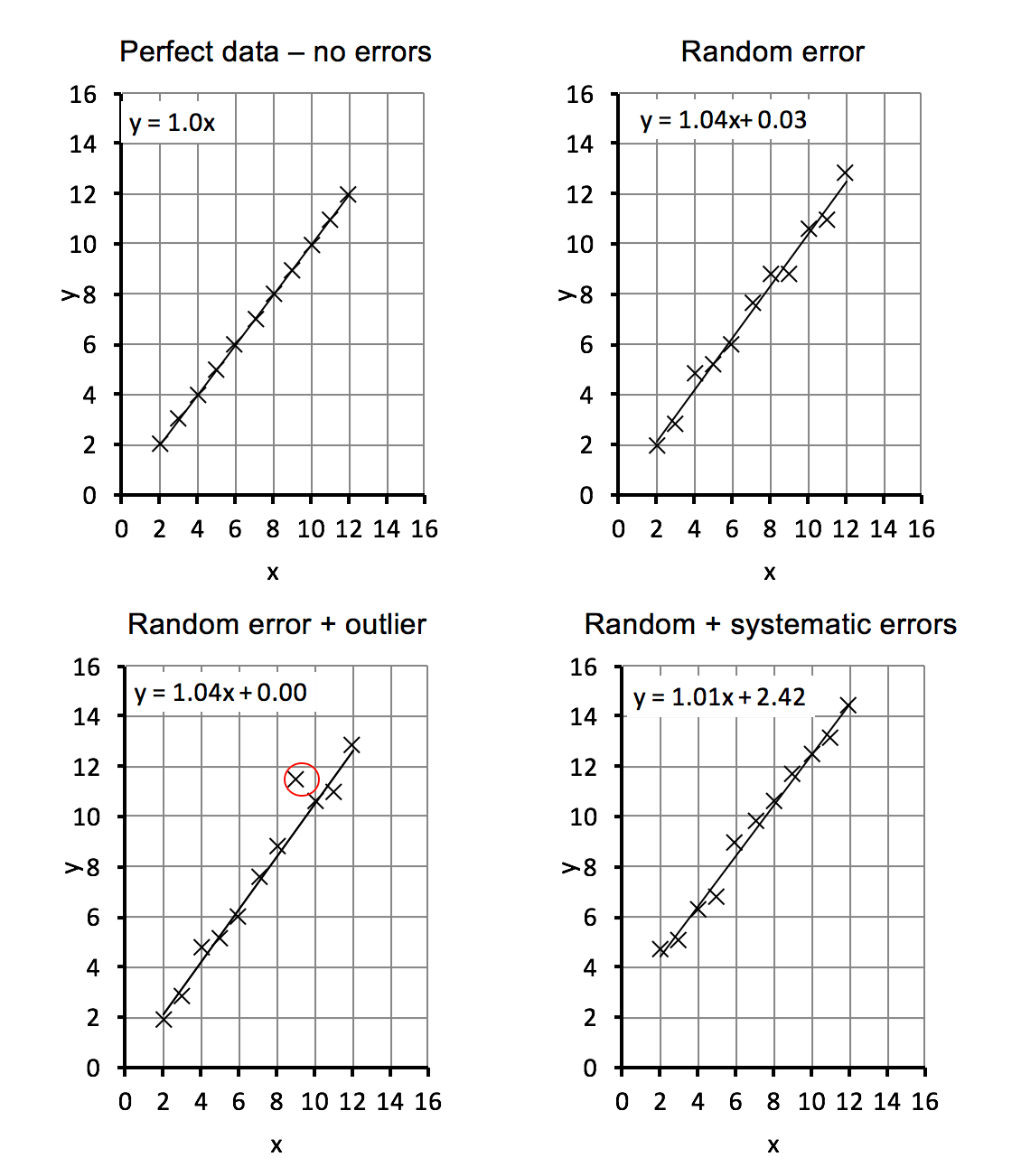
The graphs below illustrate four examples with different types of errors and with no errors:
- Perfect data with no errors
- Random error
- Random error with outlier
- Random and systematic errors
The gradient represents the result of the experiment and should have a value of 1, so the equation of the line is \(y=x\).
How does using a line of best fit reduce experimental errors?
Using a graph gives results that are as good or better than calculating from the data points directly and then averaging, as shown in the table below.
| Types of error present | Result obtained from the gradient of a line of best fit | Result obtained from calculating using all data points and averaging them |
| Perfect data – no errors | 1.00 | 1.00 |
| Random error | 1.04 | 1.04 |
| Random error with outlier | 1.04 | 1.07 |
| Random and systematic errors | 1.01 | 1.47 |
From the table, we can see that:
- The perfect data has a gradient of 1.
- The random error gives a gradient is 1.04, still very close to the correct value of 1.
- The outlier is ignored by the line of best fit, so the gradient is still 1.04, close to the correct value. Averaging over the data points would not ignore the outlier and would give a worse result of 1.07.
- The systematic error shifts up the data by the same amount but does not change the gradient, which is still 1.01. This is still very close to the correct value of 1.However, averaging over the points, in this case, gives an incorrect answer of 1.47.
Common mistakes with drawing lines of best fit
The example below shows common mistakes by students when drawing lines of best fit. The exact mistakes the students have made are listed below.
Mistake 1: Missing labels
Graph 1 shown below is missing labels:

Mistakes in this graph include:
- Missing labels for the axes
- Missing descriptive title
- The extrapolated line is not drawn using a dotted line
6 Common Mistakes HSC Physics Students Make in Exams
Mistake 2: Incorrect scale
Graph 2 shown below contains an incorrect scale:
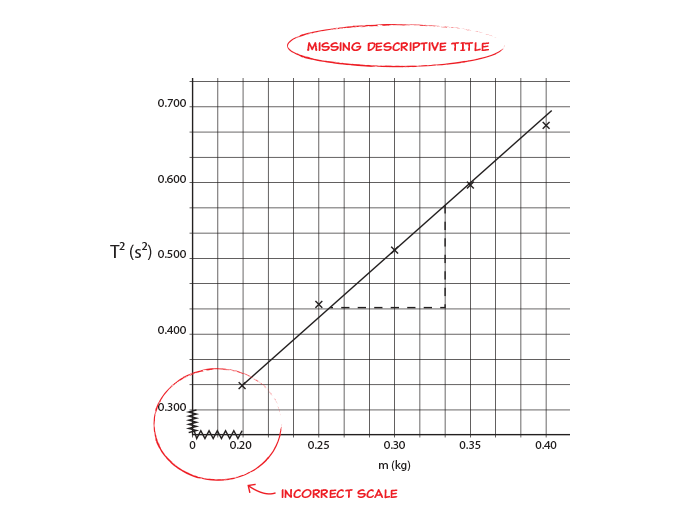
Mistakes in this graph include:
- Incorrect scale in x and y-axes. Each division should represent the same value across each axis. For example, if each division is set a value of 0.05 at the beginning of the graph for that axis, then one division should always represent 0.05 for that axis.
- The student should either start the scale at 0 or at 0.20 on the x-axis.
Want to ace your next Physics Practical Assessment?
Download the Matrix Practical Skills Workbook and sharpen your Physics skills. Learn how to:
- Assess the validity, reliability and accuracy of any measurements and calculations
- Determine the sources of systematic and random errors
- Identify and apply appropriate mathematical formulae and concepts
- Draw appropriate graphs to convey relationships

© Matrix Education and www.matrix.edu.au, 2021. Unauthorised use and/or duplication of this material without express and written permission from this site's author and/or owner is strictly prohibited. Excerpts and links may be used, provided that full and clear credit is given to Matrix Education and www.matrix.edu.au with appropriate and specific direction to the original content.
More Essential Guides
Get free study tips and resources delivered to your inbox.
Join 75,893 students who already have a head start.
How to Draw a Line of Best Fit in Maths
Source: https://www.matrix.edu.au/the-beginners-guide-to-physics-practical-skills/physics-practical-skills-part-4-how-to-draw-a-line-of-best-fit/
0 Response to "How to Draw a Line of Best Fit in Maths"
Postar um comentário